Bifurcations

Beginning with m=1 A chain of substantial changes in the system´s topology takes place.

At m=1 the Fixed points merge and exchange properties in a saddle-node bifurcation. F1 changes from a Saddle to an attractor (the positive eigenvalue changes to negative) and F0 becomes a saddle with a repelling direction towards the instable equilibrium (1/0/0/0). This is exactly the direction of the movement of F1 when m is further increased, so the homoclinic connection is established again.

An animation illustrates the saddle-node bifurcation process at m=1
A single attractor is present at (0/0/0/0) in the phase space beside of the attracting (-inf/inf/-inf/-inf) direction for m=1. The streamline starting close to the pi2 axis spirals first towards the attractor and finally esacpes into infinity.
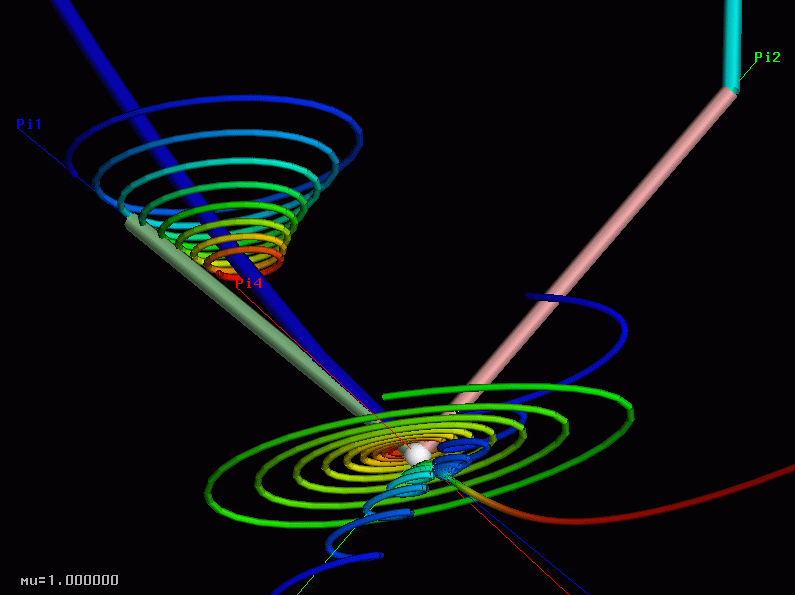Approaching m=2 the attracting and repelling components of F0 and F1 decrease and become weaker. The imaginary part of F1's conjugate complex eigenvalues goes against infinity, producing extremely fast rotation around the weak attractor.
At the same time the real part of the rotational eigenvalues of F0 reaches 0, causing F0 to become a spiral-node, a topological element not possible in less than 4D spaces! The streamlines are attracted from one side of the rotation plane and repelled from the other one. The effects of moving the startpoint close to F0 at m=2.0 are shown in this animation. The color of the streamline corresponds to the flow velocity.
With m growing larger than 2 the real parts of the complex eigenvalues of F0 become positive, system states spiral away from F0. The animation shows the effect of moving m over 2.
Above m=2 F1 appears again moving close to the stable equilibrium approaching F0 again. as a saddle with an attracting spiral and two repelling real eigenvalues.

At m=1+sqrt(3) the the real part of F1's conjugated complex eigenvalues becomes 0, causing a hopf bifurcation. During this event F1 becomes a pure repellor.

At m = 4 F1 moves to the intersection of the stable equilibrium and the pi2 axis. The complex eigenvalues of F2 change to real, rotation around it vanishes, F1 becomes a saddle with a single positive and three negative eigenvalues. Simultaneously the only attracting direction of F0 changes to repelling.